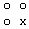2. If we wish to represent another thing, not o, we must represent it by another symbol; for we cannot distinguish between o and o except by the fact of their being spatially separated, left and right, on this page; and since this is a representation, not of a structure in space (i.e. of a spatial object), but of the structure of space (amongst other things), which structure is not itself spatial, such spatial distinctions in the representation must not be taken into account.[b] Thus, whether we write o once or a hundred times still only one thing is represented.

3. Let us, then, represent a thing other than o by x. (We are concerned to represent only the framework within which things exist, that is to say the possibility of the existence of things; consequently it does not matter whether there are in fact things -- it is enough that there could be. But the actual existence of things is indispensable evidence that they can exist; and when there actually is a given thing o, there actually are, also, other things.)[c] We now have two things, o and x.

4. We are, however, still unable to distinguish them; for, since spatial distinctions are to be disregarded, we cannot tell which is the original thing, o or x. Experience shows us that when we are conscious of one thing we are not also equally conscious of another thing; or, better, it can always be observed (by reflexion) that two (different) experiences are not both the centre of consciousness at the same time. The difference between two things is, ultimately, their order of priority -- one is 'this' and the other is 'that' --, and this difference we represent by a difference in shape; for if two things are identical in all qualitative respects, have all their properties in common (including position if they are tactile things -- and it must be remembered that the eye, since it is muscular, is also an organ of touch, giving perceptions of space and shape as well as of colour and light),[d] no priority is evident, and there are not two things, but only one; and thus difference in priority can be represented by difference of qualitative property. But difference in shape alone only tells us that if one of them is 'this' the other is 'that' -- it does not tell us which is 'this'.[e]

5. We have, then, to distinguish between first and second, or one and two. At first sight this seems easy -- one is obviously o and two is o x. But since it makes no difference where we write these symbols (spatial distinctions being of no account), we cannot be sure that they will not group themselves o o and x. Since o and o are only one thing, namely o, we are back where we started.

6. To say that o and o are only one thing is to say that there is no difference between them; and to say that o and x are two things is to say that there is a difference between them (no matter which precedes). In other words, two things define a thing, namely the difference between them. And the difference between them, clearly, is what has to be done to pass from one to the other, or the operation of transforming one into the other (that is, of interchanging them). A little thought will show that this operation is invariant during the transformation (a 'journey from A to B' -- to give a rough illustration -- remains unchanged as a 'journey from A to B' at all stages of the journey), and also that the operation is a thing of a higher or more general order than either of the two things that define it (a 'journey from A to B' is more general than either 'being in A' or 'being in B' since it embraces both: a 'journey from A to B' may be defined as the operation of transforming 'being in A' into 'being in B' and 'not being in B' into 'not being in A'). Each of these two things, furthermore, is itself an operation of the same nature, but of a lower or more particular order (a 'journey from one part of A [or B] to another' is 'being in A [or B]', just as a 'journey from A to B' is 'being in Z', where A and B are adjacent towns and Z is the province containing them). But we must get back to our noughts and crosses.

7. Since o o is one, and o x is two (though the order of precedence between o and x is not determined), it is evident that we can use these two pairs to distinguish between first and second. In whatever way the four symbols, o, o, o, and x, may pair off, the result is the same (and it makes no difference whether o o is regarded as one thing and o x as two things, or, as in the last paragraph, o o is regarded as no operation and o x as one operation -- nought precedes one as one precedes two). We have only to write down these four symbols (in any pattern we please) to represent 'two things, o and x, o preceding x'.

8. As these four symbols pair off, we get two distinguishable things, o o and o x (which are 'o first' and 'x second'). These two things themselves define an operation -- that of transforming o o into o x and o x into o o. This operation is itself a thing, which we may write, purely for the sake of convenience, thus: .

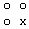9. It will readily be seen that if 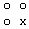is a thing, then another thing, not 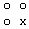, will be represented by 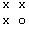; for if we take 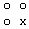as 'o precedes x', then we must take 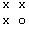as 'x precedes o'. But we do not know which comes first, 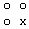or 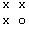. By repetition of the earlier discussion, we see that we must take three of one and one of the other to indicate precedence; and in this way we arrive at a fresh thing (of greater complexity) represented by 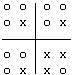. 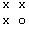Here it is clear that though in the fourth quarter, , x precedes o, yet the first quarter, 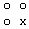, precedes the fourth quarter. So in the whole we must say 'o precedes x first, and then x precedes o'.

10. Obviously we can represent the negative of this fresh thing by 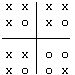, and repeat the whole procedure to arrive at a thing of still greater complexity; and there is no limit to the number of times that we can do this.

11. In §7 we said that in whatever way the four symbols, o, o, o, and x, may pair off, the result is the same. In how many ways can they pair off? To find out we must number them. But a difficulty arises. So long as we had the four symbols written down anywhere, the objection that we were using spatial distinctions to distinguish one o from another did not arise (and in §8 we noted that we chose to write them 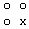purely for convenience' sake). Once we number them (1, 2, 3, 4), however, the objection becomes valid; for the only distinction between o₁ and o₂ and o₃ -- apart from the numbers attached to them -- is their relative spatial positioning on this page. But at least we know this, that 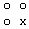represents 'o precedes x'; and so it follows that, even if we cannot distinguish between the first three, x comes fourth. In any way, then, in which we happen to write down these four symbols, x marks the fourth place. (If, for example, we had written them o x o o, the symbol x would still mark the fourth place.) And if x comes in the fourth place in the first place, it will come in the first place in the fourth place. This means that we can choose the first place at our convenience (only the fourth place being already fixed) and mark it with 'x in the fourth place', i.e. 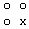. With the fourth place determined, we are left with a choice of three possible arrangements: 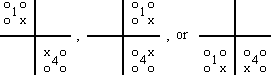. Note that we must adjust the position of x in the fourth tetrad to come in whichever place we choose as the first. Let us (again purely for convenience' sake) choose the first of these three possibilities. It is clear that if x comes in the fourth place in the first place and in the first place in the fourth place, it will come in the third place in the second place and in the second place in the third place. So now we can complete the scheme thus: 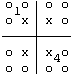. But although we can now distinguish between the second place and the third place, we cannot tell which of the two, 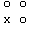or 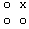, is the second and which the third: all we can say is that if one of them is the second the other is the third. This, as we shall see, is all that is necessary. Let us refer to them, for convenience, as 2/3 and 3/2, so: 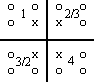. Replacing the symbols by numbers, we finally have this: 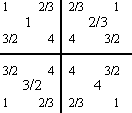(the figure is enlarged to accommodate the numerals).

12. In this way the four symbols, o, o, o, and x, when written 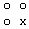, can be numbered 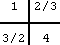; and we see that pairing off can be done in three ways: [1 - 2/3] [3/2 - 4], [1 - 3/2] [2/3 - 4], and [1 - 4] [2/3 - 3/2]. These may be understood as the operations, respectively, (i) of interchanging column with column 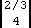, (ii) of interchanging row 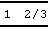with row 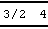, and (iii) of doing both (i) and (ii) in either order and therefore both together (this really means that the three operations are mutually independent, do not obstruct one another, and can all proceed at once).[f] And these, when set out in full -- first the original arrangement 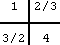(which may be taken as the zero operation of no interchange), and then the results of the other three operations, 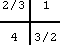, , 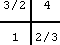and 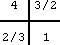 --, make up the figure at the end of the last paragraph. It is easily seen that no question of priority between 2/3 and 3/2 arises.

13. We have found that a thing can be represented, in increasing complexity of structure, as follows: o, 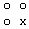, , 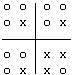and so on, indefinitely. The first of these, o, clearly does not allow of further discussion; but the second, 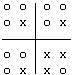, as will be seen from what has gone before, can be regarded as a combination, or rather superposition, of four operations: no interchange, interchange of columns , interchange of rows 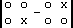, and interchange of columns and rows together 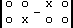; the whole being represented so: 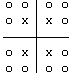. 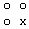A thing represented by , that is to say, consists of four members, one of which corresponds to each of the four operations. As we go to greater complexity and consider a thing represented by 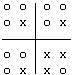, we find that the following operations are superposed: no interchange; interchange of column 1 with column 2 and of column 3 with column 4; similar interchange of rows; interchange of column 1-&-2 with column 3-&-4; similar interchange of rows; and any or all of these together. The total is sixteen; and the whole representation is given below (the numbers are not necessary but are given for clarity's sake, with 2/3 just as 2 and 3/2 as 3 and corresponding simplifications in the other numbers).

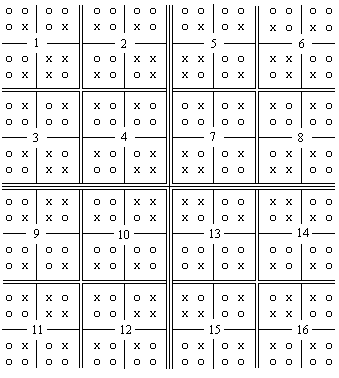
Here we have sixteen members, one corresponding to each operation (as before). If we go to still more complex representations of a thing (as indicated in §10) we shall get 64 members, and then 256 members, and so on, indefinitely. Note that any of these representations can -- more strictly, though less conveniently -- be written in one line, in which case there are no columns-and-rows; and we are then concerned throughout only with interchanges of symbols -- singly and in pairs, in pairs of pairs and in pairs of pairs of pairs, and so on. (This, incidentally, throws light on the structure of a line; for we are taking advantage of the structure of a line to represent structure in general. The structure of the line -- or, more exactly, of length -- is seen when we superpose all the members of the representation.)

14. It is a characteristic of all these representations that the operation of transforming any given member into any other member of the set transforms every member of the set into another member of the same set. The whole, then, is invariant under transformation. Attention, in other words, can shift from one aspect of a thing to another while the thing as a whole remains absolutely unchanged. (This universal property of a thing is so much taken for granted that a structural reason for it -- or rather, the possibility of representing it symbolically -- is rarely suspected.) See CETANÁ (Husserl's cube).

15. Representations of a thing in greater complexity than the 4-member figure show the structure of successive orders of reflexion (or, more strictly, of pre-reflexion -- see DHAMMA [b]). Thus, with 16 members we represent the fundamental structure of the fundamental structure of a thing, in other words the structure of first-order reflexion; whereas with four members we have simply first-order reflexion or the structure of the immediate thing. (In first-order reflexion, the immediate thing is merely an example of a thing: it is, as it were, 'in brackets'. In second-order reflexion -- the 16-member figure --, first-order reflexion is 'in brackets' as an example of fundamental structure.) In the 16-member representation, any two of the other 15 members of the set together with a given member uniquely define a tetrad with the structure of the 4-member representation; and any such tetrad uniquely defines three other tetrads such that the four tetrads together form a tetrad of tetrads, and this again with the same structure. From this it can be seen that the structure of the structure of a thing is the same as the structure of a thing, or more generally that the structure of structure has the structure of structure.[g] The 16-member representation gives the fundamental structure of first-order reflexion, just as 4 members represent the fundamental structure of immediacy, and the single member (o) represents simply immediacy, the thing.

16. The same structure, naturally, is repeated at each level of generality, as will be evident from the numbers in the figure at the end of §11. The whole (either at the immediate or at any reflexive level) forms a hierarchy infinite in both directions[h] (thus disposing, incidentally, of the current assumptions of absolute smallness -- the electron -- in quantum physics, and absolute largeness -- the universe -- in astronomical physics).[i] It will also be evident that successive orders of reflexion generate a hierarchy that is infinite, though in one direction only (perpendicular, as it were, to the doubly infinite particular-and-general hierarchy).

17. The foregoing discussion attempts to indicate in the barest possible outline the nature of fundamental structure in its static aspect. Discussion of the dynamic aspect must deal with the structure of duration, and will go on to distinguish past, present, and future, at any time, as over-determined, determined, and under-determined, respectively. The way will then be open for discussion of intention, action, and choice, and the teleological nature of experience generally.

Next section

Back to Clearing the Path - Contents

Back to Ñánavíra Thera Dhamma Page

Footnotes:

[a] An existing thing is an experience (in German: Erlebnis), either present or (in some degree) absent (i.e. either immediately or more or less remotely present). See NÁMA & RÚPA. [Back to text]

[b] See RÚPA [e], where it is shown that space is a secondary, not a primary, quality. [Back to text]

[c] All this, of course, is tautologous; for 'to be a thing' means 'to be able to be or exist', and there is no thing that cannot exist. And if anything exists, everything else does (see (a) above). Compare this utterance of Parmenides: 'It needs must be that what can be thought of and spoken of is; for it is possible for it to be, and it is not possible for what is no thing to be'. (Parmenides seems to have drawn excessive conclusions from this principle through ignoring the fact that a thought is an imaginary, and therefore absent, experience -- or rather, a complex of absent experiences --; but the principle itself is sound. The images involved in thinking must, individually at least [though not necessarily in association], already in some sense be given -- i.e. as what is elsewhere, or at some other time, or both -- at the immediate level, before they can be thought. Perhaps the method of this Note will suggest a reconciliation between the Parmenidean absolute denial of the existence of no thing, with its corollary, the absolute existence of whatever does exist, and the merely relative existence of every thing as implied by the undeniable fact of change.) [Back to text]

[d] Strictly, we should not go from muscles to spatial perceptions. Spatial perceptions come first; then we observe that whenever there are spatial perceptions a muscular organ can be found; finally we conclude that a muscular organ is very probably a condition for spatial perceptions. See PHASSA & RÚPA. [Back to text]

[e] McTaggart, I discover, (op. cit. §45) bases his version of fundamental structure on a twofold direct appeal to experience: first, that something exists, and secondly, that more than one thing exists. But this is not enough: it is essential also to see that, of two things, in so far as they are two, one is 'this' and one is 'that'. [Back to text]

[f] If we describe the three operations as 'horizontal interchange', 'vertical interchange', and 'diagonal interchange', it will readily be seen that any one of the three is equivalent to the other two done together. And since each is both the other two, it is not either of them. [Back to text]

[g] There is an old axiom: Quidquid cognoscitur, per modum cognoscentis cognoscitur -- Whatever is known, is known in the mode of the knower. This would imply that, if the mode (or structure) of immediate experience were different from that of reflexive experience, it would be systematically falsified in the very act of being known. A further act of reflexion would then be necessary to reveal the falsification. And this, in turn, would involve a further falsification, requiring yet a further act of reflexion. And so on indefinitely, with no end to the falsification; and fundamental structure (if any) would never be knowable. But we now see that the modes of immediate and of reflexive experience are the same, and consequently that any further act of reflexion can only confirm the original reflexive evidence, which is therefore apodictic. Fundamental structure guarantees reflexive knowledge of it. [Back to text]

[h] The structure of the immediate hierarchy, based on 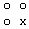, comes into view when the operations of interchange of §12 are themselves subjected to these operations. The original operations are given by 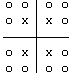, and we operate on this to get ; and, clearly, we can continue indefinitely. Similarly for the hierarchies of each level of reflexive experience. [Back to text]

[i] It is evident, in practice, that limits are encountered. There is, for example, a limit to the degree of smallness that can be distinguished. The reason for this is to be looked for on the volitional level. In order for a thing to be distinguished (or isolated) it must be observable at leisure, and this is a voluntary reflexive capacity. Beyond a certain degree of smallness this capacity fails. The smallest thing that can be distinguished has a certain appreciable size, but the visual (tactile) oscillations can no longer be controlled reflexively so that one part may be distinguishable from another part. And conversely, above a certain degree of largeness it is not possible to pass from one part to another at will, so as to appreciate the whole. Similar considerations will apply to perceptions other than size. The range of voluntary reflexion is not dictated by fundamental structure and varies (we may presume) from individual to individual, and particularly from individuals of one species to those of another. The ranges of an elephant and of an ant, at least as regards spatial perceptions, will scarcely overlap at all.

The existence of such limits can easily be demonstrated by an artificial device. If a cinematograph film is projected slowly enough, we perceive a series of stills, each of which we can examine individually. When the projection is speeded up, this examination becomes more difficult, and the series of stills is seen as a flicker. Then, at a certain point, the flickering ceases and we see simply a single (moving) picture. If, on the other hand, the projection is slowed down instead of speeded up, there comes a point past which the individual stills are no longer grasped as forming part of a series, and the unity of the film as a whole is lost. [Back to text]